![]()
The dynamics of Josephson junction is given by the nonlinear differential equation
![]()
which is the equation for the forced damped driven pendulum (Ref. [1]). This analogy is used for graphic presentations.
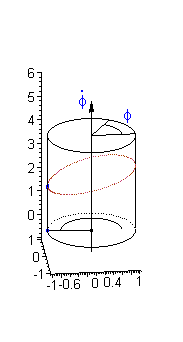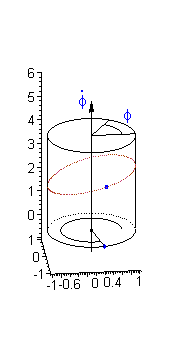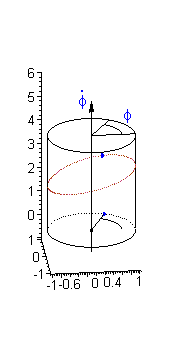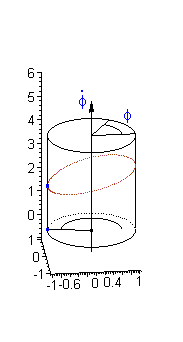
Since the unknown variable appears under sine function it is convenient to take a cylinder phase space.
 |
|
It is important to note, that in cylindrical phase space there are two kinds of closed paths:
a) Closed paths of the first kind encircling an equilibrium point. In term of the mechanical analog this type of trajectory corresponds to an oscillatory motion of the pendulum.
b) Closed paths of the second kind (see above) go around the cylinder without encircling an equilibrium point. In term of the mechanical analog this type of trajectory corresponds to a rotary motion of the pendulum.
For discrete nonlinear system into environment the closed paths of the first kind lead to the discrete breathers (DB). The closed paths of the second kind lead to the rotobreathers (RB).
For junction and damped pendulum there is only closed path of the second kind.
Josephson junction ladder
The system of equations for Josephson ladder are
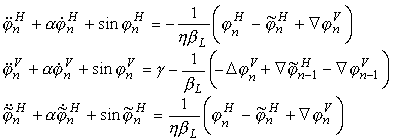
where

These equations are used for investigations of the rotobreather structures. There are different methods for obtaining of RB(DB) solutions in discrete nonlinear systems. One of the methods is realized in MapleVR5 code (JL.wms) and presented at the MapleV worksheet. The realized method, parameters and results are described in Ref. [2]. Experimental results are given, for example, in Ref. [3, 4].
Two cell rotobreathers in Josephson ladder are presented here:
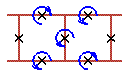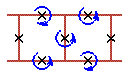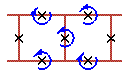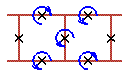 |
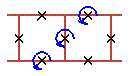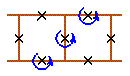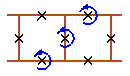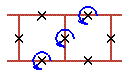 |
 |
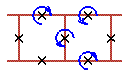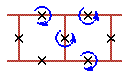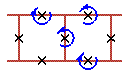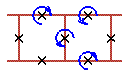 |
To see rotobreather dynamics and representation within the framework of pendulum analogy click on the picture [up-down symmetry Sud (Fig. 1), inversion symmetry Sin (Fig. 2), left-right symmetry Slr (Fig. 3), no symmetry (Fig. 4)].
An examples of three cell rotobreather structures in Josephson ladder are given here
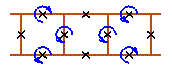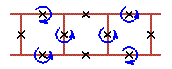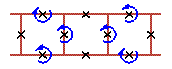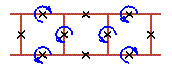 |
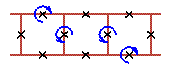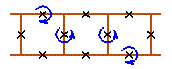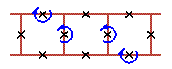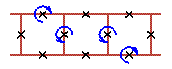 |
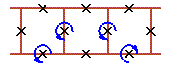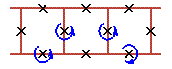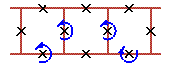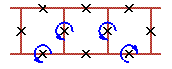 |
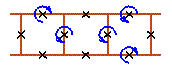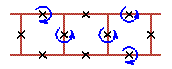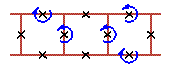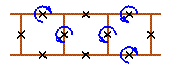 |
To see rotobreathers (Fig. 5, Fig. 6, Fig. 7, Fig. 8) click on the picture.
Many cell rotobreather structures can be derived too. To see some of them click here: many cell rotobreathers.
References
=================================
=================================
Thank you for visiting this page. You are very welcome to contribute ideas for improvements. Let me know what you think. Andrey
Link: Web Site Solitons and Soliton Collisions (Tver State University).