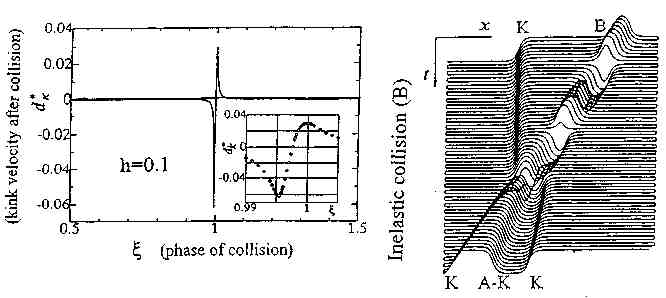
=================================================

1. The collision between a kink and a high amplitude breather in the Frenkel-Kontorova chain with a small degree of discreteness was studied numerically. The results were compared with an exact three-soliton solution to the sine-Gordon equation.

2. Even for a small degree of discreteness there exists a narrow range of parameters in which the collision is strongly inelastic.

3. The phenomena are due to the existence of a stochastic layer in the vicinity of a separatrix of the phase space of the colliding solitons. The width of the layer is not equal to zero, no matter how small is the amplitude of random perturbation.

4. Collision of solitons in a system with a small random perturbation has a probabilistic character, the result of collision is, generally speaking, unpredictable.